This one may be a little spooky.
White to play.
| Life In 19x19 http://www.lifein19x19.com/ |
|
| Late Halloween problem http://www.lifein19x19.com/viewtopic.php?f=15&t=13755 |
Page 1 of 1 |
| Author: | Bill Spight [ Fri Nov 04, 2016 8:26 pm ] |
| Post subject: | Late Halloween problem |
This one may be a little spooky. White to play. |
|
| Author: | EdLee [ Sat Nov 05, 2016 12:09 am ] |
| Post subject: | |
Hi Bill  |
|
| Author: | Bill Spight [ Sat Nov 05, 2016 1:18 am ] |
| Post subject: | Re: |
EdLee wrote: Hi Bill Hi, Ed. Hidden for no good reason.  |
|
| Author: | EdLee [ Sat Nov 05, 2016 1:34 am ] |
| Post subject: | |
Hi Bill |
|
| Author: | lightvector [ Sat Nov 05, 2016 6:23 am ] |
| Post subject: | Re: Late Halloween problem |
Solution attempt, but I didn't as thoroughly try to understand the situations involved as on past endgame problems, so I might be missing something. |
|
| Author: | Bill Spight [ Sat Nov 05, 2016 9:09 am ] |
| Post subject: | Re: Late Halloween problem |
lightvector wrote: Solution attempt, but I didn't as thoroughly try to understand the situations involved as on past endgame problems, so I might be missing something. |
|
| Author: | Bill Spight [ Thu Nov 10, 2016 10:18 pm ] |
| Post subject: | Re: Late Halloween problem |
lightvector solved the problem, as usual.   Nobody else posted an attempt, so I don't see much point in hiding this.  at   at 4This diagram shows technically correct play. White gets the last move and wins by 1 point. If White deviates, Black can make jigo.  at  at 6The hane,  , holds Black to 4 points in the corner, but then Black can get the last play for jigo.One problem with this problem is that the failure option for White is not very intuitive. OTOH, one thing I like about it is that each independent region is a little tricky. More analysis later.
|
|
| Author: | Bill Spight [ Fri Nov 11, 2016 6:39 pm ] |
| Post subject: | Re: Late Halloween problem |
As with so many of my problems, this one ultimately comes down to getting the last move that gains one point. Let's take a look at different areas of the board from that perspective. After  a move at "a" will gain one point for either Black or White. The bottom right goes into the undecided or fuzzy column.However, Black will get the last play in the top left corner.  fills at If the top left corner is the last place on the board left to play, then Black will get the last play. In the fight to get the last play, we say that it is positive for Black. OC, Black will avoid playing there, and by the same token White will wish to play there. Now let's look at the bottom left. captures the stone, leaving miai in the corner. saves the stone. Since each player can play first and get the last play, the bottom left is fuzzy, too. More analysis to come.
|
|
| Author: | Bill Spight [ Sun Nov 13, 2016 6:22 pm ] |
| Post subject: | Re: Late Halloween problem |
Let's take a look a little later in the game. Leaving A couple of things to note about this position: First, White has played with gote in the top left, but it is still positive for Black. The resulting shape (marked) is a familiar one, called an UP. (See http://senseis.xmp.net/?UP and linked pages.) In an UP, Black to play can take the last play or White to play can play to a simple fuzzy position called a STAR. (See http://senseis.xmp.net/?STAR and linked pages.) The position on the right side and the position after White captures the  stones are examples of STAR. Since White has to take gote to reach UP, the original position in the top left corner is more positive that UP. In fact, it is called DOUBLE UP STAR. (See http://senseis.xmp.net/?CorridorInfinitesimals) Second, even though Black plays first in this position, in spite of the fact that the top left is positive for Black, White gets the last play. That means that the rest of the board is negative for Black (positive for White), in fact, at least as negative as UP is positive. And that means that two fuzzy positions add up to a negative position. Most peculiar, Mama!We have already seen, without comment, how two fuzzy positions can add up to a non-fuzzy position. See next diagram. After the capture of the stone and the capture of the stone are miai. Both local positions are fuzzy, but because of miai, they add up to the same thing, 1 net point for Black, no matter who plays first. We say that two STARs add up to 0. It turns out that after  the UP in the top left is miai with the two fuzzy positions on the rest of the board. White to play will capture the stones and then Black will play  to settle the bottom left corner. The result is the same as when Black plays first. That means that the two positions in the bottom left corner and right side add up to the negative of UP, which is DOWN. In fact, the bottom left corner is worth DOWN plus STAR, or DOWN STAR, which, added to the STAR on the right, yields DOWN.More to come!
|
|
| Author: | Bill Spight [ Tue Nov 15, 2016 10:49 am ] |
| Post subject: | Re: Late Halloween problem |
Bill Spight wrote: Why do I make that claim? Well, is correct, at least if the rest of the play is. I will defend later.  and play where Black is positive, which is correct. What about  , though? Why not play at 9? OC, we could read it out, but Black loses, anyway. Why is technically correct? After all, both plays gain just a much.To answer that question we can compare the two plays with a difference game. (See http://senseis.xmp.net/?DifferenceGame ) The question is whether Black should capture the White stone in the bottom left corner or the White stone on the right side. To set up the difference game, for convenience I have moved the position on the right side to the bottom right corner. That is not necessary, but it makes the setup easier. Then I have mirrored the bottom position on the top. In the difference game play is restricted to the regions of interest, the bottom two files and the top two files. The rest is no man's land. Mirroring sets up a miai which yields jigo, no matter who plays first. The position is strictly even. To compare the plays we let Black make one of the plays on one side of the board and White make the mirror of the other play. The order of plays does not matter. White has one Black prisoner. If the difference game is strictly even, then the moves are equivalent. If the player who plays first wins the game, then we cannot say which move is better. That depends upon the rest of the board in each game. But if one player can win the difference game by playing first while getting at least a jigo by playing second, then the difference game favors that player, and that player's move is superior -- with one proviso. If there is a ko elsewhere in the real game, that can make the other play better. In this case we know that the difference game is an UP, which is positive for Black. That means that Black will get the last play and win when she plays first, and will get the last play for jigo when White plays first. So the play in the bottom left corner is technically correct. Out of curiosity, what about White's play? White has three choices: save the stone on the right side, save the stone in the bottom left corner, or make an eye in the bottom left corner, leaving the corner stone en prise. We cannot say which play is better in general, but we can show that saving the stone in the bottom left corner is technically incorrect. Saving the stone on the right side is superior. ---- Edit: I misspoke.  Actually, we can show that both the capture of the stone on the left and the stone on the right are inferior. Making a point on the bottom left and leaving both corners miai is technically correct. It does not require a difference game to show this.If saves the stone, gets the last play. This is bad for White because the position is positive for White. It is wrong for White to let Black get the last play. The same is true for this line of play. The only technically correct play is .---- To set up the difference game White saves the stone in the bottom right and Black saves the mirror stone in the top left corner. Note that White finishes off the top left corner in sente. Those three plays form a unit. If Black allows White to capture three stones she is worse off than if she had not connected to the corner stone in the first place. This difference game is a DOWN, which is good for White. So White's play of saving the stone on the right is superior, and saving the stone in the bottom left corner is inferior, and technically incorrect. Edit: The setup of the difference game is wrong, because Black will not play in the top left corner after White plays in the bottom right corner. The result is an UP, which Black wins. That shows that is incorrect.Here is an edited SGF showing play in the difference games. Even more to come!
|
|
| Author: | Bill Spight [ Tue Nov 15, 2016 1:57 pm ] |
| Post subject: | Re: Late Halloween problem |
I claim that is technically incorrect, but how does play go if Black plays there? captures 2 stones at takes back at 4If White plays this way Black gets the last play for jigo. Where did White go wrong? Black has captured 3 White stones, White has captured 2 Black stones is tesuji. Black cannot afford to play the ko, so White gets the last play to win by 1 point. Note that White cannot afford to play earlier.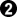 may not be technically correct, but it gets jigo if White does not see .More later.
|
|
| Author: | Bill Spight [ Fri Nov 18, 2016 11:05 am ] |
| Post subject: | Re: Late Halloween problem |
Well, it's already the day of the big match in Japan, so I'll be brief. I made a misstatement in post #10 ( viewtopic.php?p=213101#p213101 ), and have corrected it. I have claimed that in the top right corner is technically correct. That claim depends upon Black not fighting the ko in the top right corner, which she obviously cannot do in the problem. But I also think that the ko is a picnic ko, and the standard textbook treatment of picnic kos is to avoid them. OC, if Black is komaster for the ko she can play it, but it is difficult for Black to be komaster. Black has the option of playing in the bottom left corner, instead of in the top right corner. Let's compare the two plays with a difference game, even though difference games do not handle kos. I think that it will be instructive. For convenience, I have moved the bottom left corner position to the bottom right corner.For the setup we let Black play in the top right corner and White play in the bottom left corner. Both corners are settled, so the remaining areas of play are the top left corner and the bottom right corner. The rest is no man's land. is sente, threatening to kill the White group. After  protects,  gets the last play to win by 1 point. makes 1 point and threatens to save the stone, but Black plays sente in the top left and then captures for the last move and jigo. is tesuji. avoids the picnic ko, but allows Black to get the last play, for jigo. Now, if we assume that Black avoids the picnic ko, then there is no ko, and the difference game is a good guide. However, in this case White can win the ko. The ko fight is instructive.  ,  take koWhite wins the ko because he has two large ko threats. threatens to kill the Black corner outright, and  threatens ko for the corner. So it is certainly possible for White to be komaster.However, look at Black's ko threat. All it threatens is to save the stone and still make jigo. It is, I think, the fact that White needs large ko threats while Black needs only small threats that makes it hard for White to be komaster, and makes this a picnic ko. (Also the fact that it is a direct ko, neither an approach ko nor a 10,000 year ko, which also may have asymmetrical ko threats.)Anyway, my claim that is technically correct is certainly debatable. ---- BTW, I got the idea for this problem while playing around with the top right corner. This kind of thing is one reason why I think that tsumego and yose benefit from being studied together.
|
|
| Page 1 of 1 | All times are UTC - 8 hours [ DST ] |
| Powered by phpBB © 2000, 2002, 2005, 2007 phpBB Group http://www.phpbb.com/ |
|
at H-08. In fact, after