BadukStone wrote:
In Lee Changho's Selected Tesuji Problems, I've encountered a tesuji-problem which, I think, has two solutions (a and b):
Black to play
'a' was the solution given in the book, and I agree with that, but I think 'b' is also the right move.
My question is whether this problem has two solutions or not.
Black to play
'a' was the solution given in the book, and I agree with that, but I think 'b' is also the right move.
My question is whether this problem has two solutions or not.
It appears to have at least 3.
Interestingly, the Web Go Board extension that I use took your original asymmetrical 10x11 diagram and turned it into a 10x10 (a previously undiscoverd bug?), changing the problem to the situation below. This appears to have only one solution. What is it?
 (b) is wrong this time, because the throw-in at
(b) is wrong this time, because the throw-in at  works now:
works now: 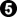 at
at