The leftmost play at A7 is 4 points gote for either side.
The next one over at D7 is 5 points gote for either side.
The lower play at G1 is 2 points gote for either side. However, if black takes it, it creates a 5 point ko threat for black.
The ko, if white fills it, saves 6 points and creates a one point sente followup. If black fills it, he takes all 7 points, but he does so over two moves, so each move is 3.5 points. However, the two left side plays are miai-ish and both more valuable than 3.5 points.
- Click Here To Show Diagram Code
[go]$$  at marked,
at marked,  at
at  White by 1
White by 1
$$ ------------------
$$ | . . . O X X . . . |
$$ | X X X X O X O X . |
$$ | 3 O O 2 O X . X . |
$$ | X X O X O X 7 X . |
$$ | O O O X O X O X . |
$$ | . . O . O X O X X |
$$ | . O . O O O 1 W X |
$$ | . . . O . O O X X |
$$ | . . . . O X 5 X . |
$$ ------------------[/go]
I suppose the crux of it is that the ko is worth less than either of the two plays on the left, but that they can be used as ko threats because they are almost miai, being a net gain of 1 point for the first side to play and more valuable than an individual move for black in the ko. Also, the total value of the two moves is equal to the total value of the ko and G7.
White has an advantage in the ko in being able to finish it in one move rather than two, as can be seen when white fills the ko for the first move and wins, but black can't take the ko first and win. So, as mentioned earlier, black has to play out the miai-ish moves on the left first. Once black starts that, though, white's play to fill the ko is 7 points vs. 4 points for the gote at A7.
Black starts out 3 points ahead without counting any of the moves, so white has to gain 4 points on black to win but has no ko threats. However, due to the ko, black is 3.5 points behind white locally, and really only half a point ahead before play commences. The key for black is to clear the ko threats first, while white needs to fill first due to the lack of ko threats.
- Click Here To Show Diagram Code
[go]$$ Best for black, victory by 1
$$ ------------------
$$ | . . . O X X . . . |
$$ | X X X X O X O X . |
$$ | 3 O O 1 O X 5 X . |
$$ | X X O X O X 4 X . |
$$ | O O O X O X O X . |
$$ | . . O . O X O X X |
$$ | . O . O O O 2 O X |
$$ | . . . O . O O X X |
$$ | . . . . O X 6 X . |
$$ ------------------[/go]
- Click Here To Show Diagram Code
[go]$$W Best for white, victory by 1\n 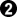 and
and  can be exchanged
can be exchanged
$$ ------------------
$$ | . . . O X X . . . |
$$ | X X X X O X O X . |
$$ | 5 O O 2 O X 4 X . |
$$ | X X O X O X 3 X . |
$$ | O O O X O X O X . |
$$ | . . O . O X O X X |
$$ | . O . O O O 1 O X |
$$ | . . . O . O O X X |
$$ | . . . . O X 6 X . |
$$ ------------------[/go]
If white lets black play at

, black can trade A7 for G1 and the ko threat is enough to win the ko later.
It's hard to use words to explain what's going on, I find. Perhaps it's best to say that every other move on the board affects the value of the ko, or that there's a value that's attached to the ko threat black can create, but it's very hard to define.
Maybe the best way is to say that the two sides appear equal, but white needs to remove the value of the potential ko threat while black needs to create it. However, playing the move that creates the threat isn't valuable enough to play so it needs to be dealt with indirectly. This seems to miss the single/double move required for the ko though.
Did I miss anything? How would you explain this?
 at marked,
at marked,  at
at  White by 1
White by 1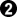 and
and  can be exchanged
can be exchanged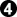 is a sente sequence, which we assume that White will be able to play. The local result is 0.
is a sente sequence, which we assume that White will be able to play. The local result is 0.  stones plus the empty point,
stones plus the empty point,  .
.