Life In 19x19

One nice thing about the yose chapter in Nogami’s book is that he includes this position.

Click Here To Show Diagram Code

[go]$$B Miai
$$ . . . . . . . . .
$$ . O O O O O O O .
$$ . . X C X C X . .
$$ . . X C X C X . .
$$ . . X X X X X . .
$$ . . . . . . . . .[/go]

Assuming that all stones are alive, Black has one point of territory among the marked points. The two short corridors are miai.

Click Here To Show Diagram Code

[go]$$W White first
$$ . . . . . . . . .
$$ . O O O O O O O .
$$ . . X 2 X 1 X . .
$$ . . X C X . X . .
$$ . . X X X X X . .
$$ . . . . . . . . .[/go]

If takes away a potential point in one corridor, 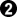makes a point in the other.

Click Here To Show Diagram Code

[go]$$B Black first
$$ . . . . . . . . .
$$ . O O O O O O O .
$$ . . X 2 X 1 X . .
$$ . . X . X C X . .
$$ . . X X X X X . .
$$ . . . . . . . . .[/go]

OTOH, if claims one point of territory in one corridor, takes away the potential point in the other.

All same same.

Now, it is true that the play may not go either way, especially in a ko fight, but it is still right to count Black with one point of territory. And it is right to count Black with 0.5 point of territory in each corridor.
(Some people have trouble believing that we should count fractional values for territory. I hope they find this kind of diagram convincing. )

Each move in the corridor gains 0.5. For a Black move, 0.5 + 0.5 = 1. For a White move, 0.5 - 0.5 = 0.

This method gives us another way of finding the value of moves. We find the value of the initial and resultant positions and take the difference.

Make the corridor one longer and it takes 4 of them to make a strict miai, that is , one with a definite score.



Quick! How many points of territory does Black have in the four corridors?

Answer in the SGF file and hidden next.
How much does a play gain in each corridor?
This method of calculating territory by play in a number of duplicates of a position is developed on Sensei's Library at http://senseis.xmp.net/?MethodOfMultiples . The fact that you can make a strict miai of some number of these positions indicates that they are gote.

Bill Spight wrote: And it is right to count Black with 0.5 point of territory in each corridor.
(Some people have trouble believing that we should count fractional values for territory. I hope they find this kind of diagram convincing. )

Each move in the corridor gains 0.5. For a Black move, 0.5 + 0.5 = 1. For a White move, 0.5 - 0.5 = 0.

This highly depends on your definition of "right".It is definitely the best practical choice and gives the best estimate you can find in reasonable time. But If there is an odd number of such half points, the "right" count giving the result, that pefect play would lead to, would be rounding the last one up for the player who "should" end up with tedomari assuming perfect play. But of course, that is no help, because if you know, what perfect play looks like, you dont need to count the current position, just count the resulting final position. It is basically the same thing as the question, whether 6,5 komi is "right". In one sense it is, because in practice it works well and leads to even games, but in another sense a fractional value cant be the right answer to the Question "how big is blacks starting advantage with perfect play?"

Schachus wrote:

Bill Spight wrote: And it is right to count Black with 0.5 point of territory in each corridor.
(Some people have trouble believing that we should count fractional values for territory. I hope they find this kind of diagram convincing. )

Each move in the corridor gains 0.5. For a Black move, 0.5 + 0.5 = 1. For a White move, 0.5 - 0.5 = 0.

This highly depends on your definition of "right".It is definitely the best practical choice and gives the best estimate you can find in reasonable time. But If there is an odd number of such half points, the "right" count giving the result, that pefect play would lead to,

The count is not the same as the score, except at the end of a scored game. So the right count does not necessarily give the result of play, perfect or otherwise.

But of course, that is no help, because if you know, what perfect play looks like, you dont need to count the current position, just count the resulting final position.

Indeed. If you know perfect play you do not need any theory.

It is basically the same thing as the question, whether 6,5 komi is "right". In one sense it is, because in practice it works well and leads to even games, but in another sense a fractional value cant be the right answer to the Question "how big is blacks starting advantage with perfect play?"

No, it isn't. (Not that I knew that, way back when.) Komi may be an estimate of the final score. The count, before the end of play, is an estimate of the current value of the board. But the values of independent positions are not just estimates. They add up more precisely than mere estimates. For instance, suppose that we end up with a board where the only plays worth playing are simple gote, each of which gains 0.5 on average. Suppose that there are, to pick a number, 25 of them. What is the expected difference between the count and the final score, given correct play? 0.5*(√25) = 2.5 ? No. It would be 0.5, the same as with one of them. If there were 26 of them, the error would be nil. We could score the position without further play. (The rules might require further play, but it would be pro forma.)

The books I read did not show the miai of those four corridors. But what they did show, and what almost every yose book shows, is the set of progressively longer corridors.



Correct play is in the long corridor. The longer the corridor, the closer the gain from the move approaches 1.

What none of the books mentioned is that after the first correct incursion into the longest corridor, the resulting position is strict miai. As the SGF file shows.

At the time I regarded the count as a mere estimate. Also, I adopted a probabilistic semantics for sente and gote. If a play was gote, I took it as a 50:50 chance that either player would play it. If it was sente, I assumed that it was played in sente. (Double sente does not fit this scheme, but that did not bother me at the time. I regarded taking double sente as a free lunch. )

Thus, for an empty 4 point Black corridor I figured that Black would seal it off for 3 points 1/2 of the time, that White would play and then Black would seal it off for 2 points 1/4 of the time, and that Black would get 1 point 1/8 of the time and 0 1/8 of the time. That yields an expected value of 1.5 + 0.5 + 0.125 = 2.125 points of territory, which is correct. That approach allowed me to calculate values pretty easily.

These days I say that taking a sente play in sente has a probability of 1 - ε , to allow for the occasional reverse sente.

OK, it's high time to talk about the latest sente study. BTW, this is the kind of thing I was doing when I was studying kos. The studies overlapped.



By comparison with the previous study, White has 0.5 point less, so that Black starts with a half point advantage. This also means that the sente does not have a standard, average environment with the same temperature as the reverse sente gains.

As you can see from the SGF file:

With best play Black to play wins by 2 points.

With best play White to play wins by 1 point.

Black's best initial play is the sente.

White's best initial play is the gote instead of the reverse sente.

We'll discuss this more after tackling this next study.



Enjoy!

I'll give it a shot...



I don't fully understand this "gain" methodology but simply try to imitate Bill's calculations, crediting reverse sente with the full swing value and gote moves with half. I think I understand the "sente gains nothing" part --- it's almost tautological, in that the player is assumed to get their sente, so they gain nothing because it already belonged to them.

Thanks, Sennahoj!

Sennahoj got it right, OC. With best play Black wins by 1; with best play White wins by 1.

When the move at C gained 1 point, each player was indifferent between playing at A or B. When the move at C gained 0.5 point, neither player wanted to get it. Black took the sente at A and then the gote at B, leaving White with C. White took the gote at B, leaving Black the sente at A (which gained nothing) and C. In this case the move at C gains 1.5 points. Does this mean that each player wants to get it? Well, White does, anyway. White plays the reverse sente at A, yielding B to Black, and then gets C. If White took B first, then Black would take the sente at A and then get C, as in the previous case.

In all three cases Black can get the optimal result by taking the sente at A. In fact, as I now know, if there are no kos involved, when the choice is between a simple gote and a simple sente with a threat larger than the gote, it is not wrong to choose the sente, regardless of what else is on the board. By not wrong I mean that taking the sente instead of the gote will lead to the optimal result, given best play otherwise.

But way back when I was first studying these things, I came to believe that Black should take the gote in this and similar situations.

Below I have amended Sennahoj's SGF file to make Black taking the gote the main line, and I have added White's mistake of taking the gote as a variation.



The point of Black taking B first is that it gives White the chance to make the suboptimal response of taking A, which gives C to Black. In that case Black wins by 2 instead of 1. Yes, the reverse sente at A gains more than the gote at C, but C is the last play. White wants to get it as long as it gains more than half of what A gains.

With C so large, this example is really about who gets C. White to play gets it by taking the reverse sente at A. When Black plays first, White can always get C with correct play, but Black can lay a trap by playing B first, tempting White to make the largest play at A and letting Black get C.

Bill Spight wrote: The point of Black taking B first is that it gives White the chance to make the suboptimal response of taking A, which gives C to Black. In that case Black wins by 2 instead of 1. Yes, the reverse sente at A gains more than the gote at C, but C is the last play. White wants to get it as long as it gains more than half of what A gains.

This looked very confusing to me at first, but I guess it's like this: when white is choosing between A and C, since there are no other moves left, the convention of crediting the reverse sente with the full swing value is kind of "wrong" --- white is really choosing between a 2 point gote (A) and a 3 point gote (C), so of course C is better.

Sennahoj wrote:

Bill Spight wrote: The point of Black taking B first is that it gives White the chance to make the suboptimal response of taking A, which gives C to Black. In that case Black wins by 2 instead of 1. Yes, the reverse sente at A gains more than the gote at C, but C is the last play. White wants to get it as long as it gains more than half of what A gains.

This looked very confusing to me at first, but I guess it's like this: when white is choosing between A and C, since there are no other moves left, the convention of crediting the reverse sente with the full swing value is kind of "wrong" --- white is really choosing between a 2 point gote (A) and a 3 point gote (C), so of course C is better.

Perhaps this kind of situation is where the saying that the last play is worth sente came from. From that perspective the comparison is between a 2 point reverse sente and a 3 point "honorary sente".

Edit: But note that that saying does not apply when you are comparing gote with gote, or when you are comparing gote with sente instead of reverse sente.

The Method of Multiples for sente

Here we have four sente corridors where White threatens to save three stones. If White plays first Black gets 24 points of territory. If Black plays first she gets 25 points. We can add more duplicates of the corridor, but Black will always score one point better if she plays first. The multiple corridors never become miai. Each additional corridor adds 6 points to Black’s score, so that’s the count for each corridor. OC, there is the possibility on any given board that Black will get the reverse sente.

In this case it is obvious that these are White sente positions. However, suppose that we were not sure. We could play them out as gote and compare the results to playing them as White sente. One variation in the SGF file shows that Black does worse if the corridors are played as gote. So Black should answer White’s plays.

A curious position

Click Here To Show Diagram Code

[go]$$ Outer stones alive
$$ . . . . . . . . . . . . . . .
$$ . O O O O O O X X X X X X X .
$$ . O X X X X . . . O O O O X .
$$ . O O O O O O O X X X X X X .
$$ . . . . . . . . . . . . . . .[/go]

This position is unlikely to ever occur in a real game, but how do we count it, and how much does a play in it gain?

It is pretty obvious that by symmetry it has a count of 0. But let's apply the method of multiples.

Click Here To Show Diagram Code

[go]$$B Black first
$$ . . . . . . . . . . . . . . .
$$ . O O O O O O X X X X X X X .
$$ . O X X X X 3 1 . O O O O X .
$$ . O O O O O O O X X X X X X .
$$ . O X X X X . 2 4 O O O O X .
$$ . O O O O O O X X X X X X X .
$$ . . . . . . . . . . . . . . .[/go]

After the position is miai. and can be interchanged, and the net result will still be 0.

Click Here To Show Diagram Code

[go]$$W White first
$$ . . . . . . . . . . . . . . .
$$ . O O O O O O X X X X X X X .
$$ . O X X X X . 1 4 O O O O X .
$$ . O O O O O O O X X X X X X .
$$ . O X X X X 3 2 . O O O O X .
$$ . O O O O O O X X X X X X X .
$$ . . . . . . . . . . . . . . .[/go]

If White plays first, the net result is still 0. The original position is miai, with a count of 0 in each corridor.

Click Here To Show Diagram Code

[go]$$B Gote
$$ . . . . . . . . . . . . . . .
$$ . O O O O O O X X X X X X X .
$$ . O B B B B . 1 . O O O O X .
$$ . O O O O O O O X X X X X X .
$$ . . . . . . . . . . . . . . .[/go]

How much does gain? After it is played, the resulting position is clearly gote. Black has 9 points on the right and the result on the left will be either 0 or -8, so its count is -4. The count for the whole position is 9 - 4 = 5 points for Black. Since the original position has a count of 0, gains 5 points.

Double sente? Or not?



The play is pretty obvious. But is the position on top a double sente or not?

I guess it depends on what else is on the board... here is a modification where it's definitely not a double sente.



In this version, the gote is between the gain from the "double sente" and the its follow up:

Sennahoj is right. Whether that play is double sente or not depends upon the rest of the board.

At the same time, we know that it is a gote that gains 5 points. Because the reply gains 4 points, it will often be the case that it may be played with sente by either player. But that has to do with the whole board, not with the basic nature of the play.

In Sennahoj's first example, the two plays are miai, not just because they are the same size, but because it does not matter to the result of the game which one Black gets and which one White gets.

Below I have modified Sennahoj's second example by adding a Black stone. The count becomes 0 and both plays are the same size. However, which one to play depends upon the rest of the board. In this case, with nothing else on the board, Black to play will prefer one of the two plays and White to play will prefer the other.



Can you tell which play which player prefers, and why?