Yes, exactly correct!
A few minor comments:

O Meien and others prefer to calculate the value of a single move, rather than the difference between a pair of moves (B first versus W first), so they divide your result by two.

If the move is truly sente for W, the probability of W playing first becomes 100%, as does the probability of B answering, so the value of the starting position is 1, and the value of the move is 0 or meaningless.

If a W play here would be sente, but it is B turn to play, then for B to play here first is reverse-sente, and the value of the move is estimated as twice the normal gote value.

This, and mitsun's description of it, apply for a local gote with one player's gote follow-up.

Bill: I know you've read the book so this is for those who haven't. The reason I expressed myself the way I did is that O said (about a third of the way deep into the book):



I think the "value of the move" is what he is really relying on, not reverse sente.

Later on he says:



As you have yourself repeatedly remarked, one of O's strengths is that he defines privilege. I think the quote above shows he is relying on that and not on reverse sente.

Later still, under the heading "(2) The relationship between privilege and reverse sente," he adds:



So, again, he is bigging up privilege and downgrading reverse sente.

Again mainly for the benefit of others but also to query your statement that O "does not define sente", he says



(And obviously he talks about it a lot elsewhere, in particular in a section headed "The right timing to exercise a privilege").

Going yet deeper into the book (which is what I mean by "in passing" - it's not his starting point), O finally gets heavy on reverse sente when he says:



This ordering of ideas means more to me than anything I'd read before.

The reason I belabour this point is on behalf of people like daal and myself. O does not speak in mathlish. Mathlish is not just a dialect with different words. It's a different grammar, with a different way of ordering things. The people who mainly speak about boundary plays and counting here order things differently from daal, myself and O. The fact that people like yourself also understand O (and no doubt far better than I can) and also understand English does not mean you are not reverting to mathlish when you speak to us.

Here are a couple more of the ways of expressing himself that made O appealing to me. What he said is old hat to you. To me it was as if someone opened the curtains and let the daylight in.





I do not wish to imply any nastiness, snobbishness, arrogance or any other vice on the part of mathlish speakers. In fact people like yourself do make a genuine effort to connect with us. It works in part. dfan has been the clearest to me, and you are close behind. But I'm afraid there are others (e.g. RJ) who jump on what you say and speak entirely in mathlish, you respond - in mathlish - and us poor unilinguists are suddenly left floundering again. It's quite rare in Japanese to have people writing mathematically about go. Here the mathematicians seem to predominate.

O (in Japanese) was the guy with the lifebelt for me. If anybody wonders why I have made no effort to have O's book published in English despite having already translated it in full, the brutal answer is that westerners have made their own book market what it is. I can't really go to O and say (truthfully, based on actual experience): "this is a great book and we need it - you'll sell as many as 200 copies in five years!!! Order the Merc now!

PS As an example of English vs mathlish, take the following from earlier in the thread:



This is stripped bare of extraneous words. There's lots of if..then.. Everything is said just once, reduced to an algorithm. O, in contrast, uses (apparently) redundant words, repeats things, goes off mid-stream and tells stories, uses LOTS of diagrams, gives definitions without worrying whether they are watertight or what peers might think of them. O serves his coffee in nice cups, with milk and sugar and a slice of cake and a bit of chat. The mathlish speakers just inject the caffeine straight into the vein.

You assume that everybody would always use their specific degree of Mathlish but that is not so. A person can vary the degree from informal English via weak or strong Mathlish to formal mathematics.

In the context of degree of Mathlish, you emphasise O as somebody using lots of diagrams to explain something. However, I tend to use more diagrams although my average degree of Mathlish is higher. There is no identity between degree of Mathlish and numbers of diagrams.

You advertise your preference for a low degree of Mathlish but do not expect everybody to become a couch potato. For calculations and evaluations involving numbers, a high degree of Mathlish and mathematical annotation have the great advantages of clarity, accuracy and easy access to verification of correctness. Furthermore, variables and equations allow generalisation and recognition of application of general methods at a glance. When different kinds of numbers are calculated, the consistent naming of variables helps. Not by chance do we use T for the temperature in physics or T for the temperature in endgame theory.

One of the worst achievements of some culture is appreciation of natural language versus scepticism of mathematical language. Its clarity is an advantage - not something to be ashmed of. Correctness of move values is something to be proud of.

Well, good. I was starting to doubt my own sanity, and possibly that of others.

But I only looked at terminal positions, and as far as I can tell so did you, and I explicitly asked for an example where that is not possible, since Bill claimed that in general you can't.
Yeah, OK, I had gathered that the point values come out as half of what you'd expect. What I'm trying to figure out is - why the emphasis on position values? What is being communicated when, for example, Bill says the value of A in the original position is zero? I'm assuming there has to be some deep insight because on the face of it it's just doesn't sound very helpful.
It still seems strange to consider sente moves as having size zero. How do you compare them? The thing about reverse sente is of course standard and comprehensible to even someone like me who gets his endgame foundations from GSatE.

You really don't get it, do you, Robert?

It's NOT clear - to us. That's the whole point. It's only clear to you because there is a whole lot of axioms, principles, mathematical grammar, modes of expression, jargon, abbreviations and so on behind it that you mathlanders understand. We lesser mortals haven't got this apparatus and don't want it, not because we couldn't learn it but because we haven't got the time or the need to learn it.

I could say something to you in Japanese. It would be totally opaque to you but it would be totally clear to me and other people who knew that language. That doesn't mean you can't learn Japanese, but you've already told us many times you haven't got the time, the need or the motivation to learn it. The result is you haven't got the apparatus to understand it. I respect that and therefore speak to you in English. But you talk to me in mathlish. Why? That's rude.

It may, in context, be rude, but speaking mathlish is nothing to be ashamed of, no more than speaking Japanese is shameful, and no-one has said it is. What is shameful is stubbornly not listening to other people when they say what you say is hard to follow because it's in a "foreign" language.

John,

"rude", "stubbornly not listening": I do not join personal meta-discussion.

For almost all of us, mathematical annotation / language per se is not a foreign language because we have been taught it at school. You do understand it when reading mathematical calculations in O's book, programming your programs or posting programming exercises (in your days on rec.gamesgo).

What learners of endgame evaluation do, at first, not understand is the terms, ways of calculating specific values and all purposes of using them. When I started learning it, I had very similar problems of understanding as daal or you may have now. With a very great difference: I needed years while daal and others learn within days. Didactics and input from several sources of varying degree of (in)formality have improved. "whole lot of axioms, principles, mathematical grammar, modes of expression, jargon, abbreviations and so on" do not prevent fast learning nowadays, nor your spread of fear about them.


As a sign of resistance with mathematical annotation, you have used two types of brackets for an expression to be calculated. Are you proud of introducing superfluous confusion?

Presumably you want to oppose new terms as being "jargon", such as "the count" (of a local position). You have invested very much more time in studying traditional go terms, such as for shapes. I cannot take your reason seriously because there are only relatively very few central terms for endgame evaluation. With the pretended little time you want to spend, learn those terms that save you time.

Count is such a term. Yes, using it requires learning once its meaning "value of a local endgame position" and admitting that "value of a position" creates confusion because the ordinary meaning of position refers to the whole board position, and local position is still wrong because it does not necessarily refer to all stones and intersections shown in a diagram but more specifically to those of a local endgame.

Follower is another such term. Not only is it shorter than "follow-up position" so that also compounds can be shorter, but it is not just some follow-up position, not necessariily the one after move 1. A follower can be a follow-up position with extra properties, such as being settled. Using the term reminds us having awareness of them. We do not want to waste very much time on all follow-up positions but we want to save very much time by focusing on the relevant followers.

You spread fear for principles to be learnt. Beware of the principle of usually playing moves in order of their decreasing values. It might save you very much time so beware of the short time of having to learn it.

bernds,

in the go game, we have positions and moves. Each move transforms a position into another position. Therefore we study properties of positions and properties of moves. In endgame evaluation, we study values of (local) positions aka counts and values of moves. When studying a single move, it transform the count of the preceding position into the count of the resulting position and the considered value of the move is its gain. The gain expresses the change in counts caused by the move. When relating Black's move or sequence started by it to White's move or sequence started by it, we consider another kind of values of moves, the move value.

Positions, moves and their values are all related. Therefore we consider them all. In particular, gains and move values are derived from counts.

What is the result of a sequence? The count of the resulting position. We are still interested in its value so we do not discard it just because of having calculated the move value of the already executed move. For decisions among different moves, we would compare different resulting counts.

Already for these basic applications, counts are essential.

There is ability and there is motivation. Myself I'm a mathematician and can be expected to easily follow the discussion but I don't because precise endgame calculations do not interest me. I suspect you are interested in O Meien's endgame treatises and its impact on the world of (professional) go from a cultural perspective. When required to acquire "mathlish" to understand the technical discussions, you lose your motivation. I don't think my intellectual capabilities to acquire the lingo used here are substantially higher than yours. I think your motivation is substantially higher than mine and so I agree with Robert that you will find it quite easy to acquire the vocab of "count" or "follower".

I recently studied Remi Coulom's paper that contributed to the AlphaGo shockwave, not because I have a predisposition for computer science, but because I was motivated to understand more about machine learning and the inner working of Leela Zero. There's a big amount of "complish" in there but I persevered.

Incidentally, I don't second your comparison with Japanese. Other languages substitute each other. Math, mathlish or complish are extensions of English, necessary to convey the subtleties of the domain. Your desire to acquire expertise on the endgame through common English maybe essentially a frustrating one. I'm reminded of poor old Galilei's writings, which didn't have algebra at their disposal yet. Today, 14 year olds with only a fraction of Galilei's brain are better at expressing his laws to their peers than he was to his, because they have all acquired the language (algebra) without too many quibbles (well, ok).

Thank you for this comprehensive reply. But truth to say, I have not read the whole book. I mean, at this point, please. The last yose text I read in toto was Kano's Yose Dictionary. And I made a mistake, as you point out. O did define local sente. Incorrectly, but close enough.



Yes. Typically yose texts begin with sente and gote, which are and remain ill defined. One reason for that is that they need those concepts to determine the territorial count of non-final positions. Sente and gote positions have different characteristics, which lead to different ways of determining their territorial counts.



You end up with a kind of conundrum. You understand sente and gote in terms of the value of one move. But you find the value of one move by comparing the territorial counts of two positions. And you cannot find the territorial counts of positions without understanding sente and gote.

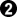
There are a couple of ways out of this conundrum. But first, let me say how O deals with it. First, he starts with gote positions. They are the easiest to understand. Later, on p. 43, he shows a sente position (Q). He shows a sente sequence in that position, - (Diagram 42), and say that 利いています。(IMO that's go jargon, and an OK translation is is forcing.) O relies upon the notion of sente but doesn't call it that.

One way out of the conundrum, which I discovered back in the '70s, is to start off assuming that everything is gote, until you discover that it isn't. What you find is that the reply to a move is larger than it would be if the move were gote. One way to describe that is to say that the initial move raised the local temperature. Now, raising the local temperature is not enough to make a play sente. Play continues until the temperature drops below what it was to start with. (How to determine that: You keep making the assumption that each play in the sequence is the last, and keep going if you can show that it isn't.) If the last play in that sequence of play was by the second player, that sequence is sente. If the last play was by the first player, the sequence is gote. (We'll get around to ambiguous later. ) Edit: You cannot decide whether a play is sente or gote without considering the other play, which will be either gote or reverse sente. O does not do that for that position.

Perhaps your eyes, or the eyes of other readers, glazed over when reading that. I submit that that is not the result of mathlish, even if the term temperature is unfamiliar. The thing is, sente is not an easy concept. We have an intuitive feel for sente which is usually right, and that is good enough for most practical purposes. The difficulty with sente is not mathlish, it is inherent in the concept. (The concept would not be difficult if there were no long sente or gote sequences.)

Another way out of the conundrum was discovered by mathematicians in the mid-20th century, in relation to evaluating game positions in general, called the mean value theorem. The go concept of miai fits perfectly into the mean value theorem. If you have some number, N, of a position such that the total score, S, of all of them together is the same, regardless of who plays first (they are miai), then the average value of each of them is S/N. That works for gote positions. The only yose text I have seen that makes use of miai in that way is in Nogami and Shimamura's book, 囲碁大観. Only for two positions, but it's a start. For sente positions and some gote positions you don't get miai, but you can show that adding a certain number of positions, M, often just one, increases the total score by a constant, C. We can take C/M as the average value of each position. This is what I call the method of multiples. An article using this method was published in the Deutsche Go Zeitung in the 1990s or early 2000s. The author consulted with me on the article.

I don't know if that caused any eyes to glaze over, but the concept of sente is a bit less difficult. Multiple copies of sente positions do not form miai. That's not all there is to it, however.

There is another way out of this conundrum, discovered in the 1970s by John Conway: thermography. Thermography is a practical, graphical way of finding both the value of a game or game position and the value of a move from that position at the same time. In 2000 I presented a paper that defined sente and gote in terms of (non-ko) thermographs. It's easy. Here is a gote thermograph.



The left wall, colored blue, indicates the result of minimax play at each temperature when Black plays first. Since Black takes gote near the top, the more that the reply in the environment gains, so the net result is less. That's why the line inclines to the right.

Similarly, the right wall, colored red, indicates the result of minimax play at each temperature when White plays first. White also takes gote, and so the right wall inclines to the left.

When the two lines meet, neither player makes a net gain from playing first, and so they are indifferent between taking the gote or not. At higher temperatures neither player will want to take the gote, which the black mast indicates. The mast rises vertically forever.

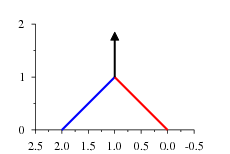
Here is a sente thermograph.



This is a Black sente. Because White replies to Black's sente, the left wall is vertical near the top. But when White plays the reverse sente, Black does not reply and White takes gote, so the right wall looks the same as that of a gote thermograph near the top.

In addition, the mast is colored blue up to temperature 4, in this case. It takes its color from the fact that the reply to it has a higher temperature than the position itself. The color represents the privilege of sente, i.e., the fact that Black will normally be able to take the sente before White is able to play the reverse sente. If the mast is not colored, then the position is ambiguous.

Back in the '90s I had hoped that by now yose books would routinely contain at least one thermograph or two. {sigh}

Enough! For now, anyway.

Edited for correctness and, I hope, clarity.

_________________
The Adkins Principle:
At some point, doesn't thinking have to go on?
— Winona Adkins

Visualize whirled peas.

Everything with love. Stay safe.

I once read some translations of what are now considered simple algebra problems from Arabic texts of the Middle Ages. They would start like this: "Heap, it's third, . . ." Mathlish makes it so much easier.

_________________
The Adkins Principle:
At some point, doesn't thinking have to go on?
— Winona Adkins

Visualize whirled peas.

Everything with love. Stay safe.

Please go back and answer the questions I posed about what you meant by certain phrases. If you do, I think that you will find that we are in agreement.



Please go back and answer my questions and I think that will become clear to you.



The not very deep insight is that if a position is symmetrical for both players, its average value is 0. If you doubt that, then flip the colors of the stones. Is it helpful? Well, it seemed to be news to daal.

Edit: Are you are still under the impression that I was talking about a play at A? If so, please reread what I wrote.

_________________
The Adkins Principle:
At some point, doesn't thinking have to go on?
— Winona Adkins

Visualize whirled peas.

Everything with love. Stay safe.

Oh, yeah?

Maybe bernds was concerned with one side having 8 and the other side having 6 points; why would you get the average 0. Answer: choose a small locale in which the hane-connect endgame is counted; its average is 0; only afterwards add the extra points outside the locale. We do so because the hane-connect shape is always the same so we can recall its count 0. It does not matter whether the settled parts of the adjacent territory regions have different shapes in different occurrences of hane-connect.

To continue, after a breather.



It seems to me that O does speak in mathlish. That is, he introduces technical terms and defines them. As is appropriate to his audience and the purpose of his book, he does not delve into the difficulties that Robert, moha, myself, and others do in our discussions here. It is not mathlish per se that makes these discussions difficult, it is their subject matter.



Sounds like mathlish to me. How does he define deficit, what does he mean by disregarding sente and gote? And anyway, the general question of when to play gote vs. reverse sente is difficult.



I think that there may be cultural reasons for that. Without a long history in the West, ordinary people are not drawn to go. Hippies were, for a while, until, as a friend pointed out, they actually had to think. For some reasons, mathematicians were, as far as I can tell. In my case, despite being fairly strong for a Western amateur, and having written a go newsletter way back when, I cannot claim any real go expertise. But in the mathematics of go, I am a world class expert.



I confess I am not quite sure what is meant.

_________________
The Adkins Principle:
At some point, doesn't thinking have to go on?
— Winona Adkins

Visualize whirled peas.

Everything with love. Stay safe.

I think he was talking about daal's original diagram and question.

_________________
The Adkins Principle:
At some point, doesn't thinking have to go on?
— Winona Adkins

Visualize whirled peas.

Everything with love. Stay safe.

Aye.

A bit more about O sente and reverse sente.



Privilege is part of the definition of local sente. In terms of thermography, it is what colors the mast. Also in terms of thermography, we can see that the reply (the next move?) is bigger than the local temperature. But the local temperature is determined, not by the size of the move just played, but by the size of the reverse sente. That is the true relationship between the reverse sente and the privilege.

On its face this definition is somewhat harder to understand than the one O gives. (But at least he gives one! ) But if you start with the simple examples that he uses, it is not difficult to convey. The point is that a sente play by you gives your opponent the chance to make a larger local play than if you had passed or tenukied. That's the right comparison to make.



Note that O has switched to a different meaning of sente. And things are not as simple as he makes out. But a little hyperbole is acceptable. And O is right that the general question of whether to play a gote or reverse sente is difficult. For daal's question of when to play a gote or sente, I said that there was good news and bad news. I can show that for the prototypical examples of gote and reverse sente, there is bad news and more bad news. That is, it will very seldom be the case that you can with certainty decide which to play without considering the rest of the board. How much each play gains is only part of the picture. (You can use it as a heuristic, but O wants to do more than that.) Considering the temperature of the rest of the board, aside from those two plays, is a little better. After that, you need to read.

_________________
The Adkins Principle:
At some point, doesn't thinking have to go on?
— Winona Adkins

Visualize whirled peas.

Everything with love. Stay safe.

Just to be sure: when you write "privilege" in your previous message, you are using it like O - as a substitute word for "sente [move of a sente sequence of a local sente]"?

By the privilege of a sente I mean that in an ideal, rich environment the player with sente will be able to play it (with sente) before the opponent will be able to play the reverse sente. (Correct play understood.)

----

For those who may be unfamiliar with the terms. An environment is the rest of the board aside from plays which are in focus. An ideal environment is one in which the largest play is always correct. A rich environment contains many plays of various sizes such that, if it is important that a play of a certain size exist, it does.

For instance, privilege requires that there be a play that is smaller than the reply to a sente, but greater than the reverse sente.

OC, no go board has an ideal, rich environment, but as a rule real boards are good approximations. If not, nobody would ever have come up with the idea of privilege.

_________________
The Adkins Principle:
At some point, doesn't thinking have to go on?
— Winona Adkins

Visualize whirled peas.

Everything with love. Stay safe.