Gérard TAILLE wrote:
Bill Spight wrote:
If Black has no ko threats, how does Black win the ko?
There are cases where the komaster will allow the koloser to win a ko, what I have dubbed
tunneling.

Why do you want black win the ko ?
For me the black strategy is different : depending of the environment black may use the following strategy : she provoques the ko, then she loses the ko but gains in exchange some points in the enviroment.
Look at the following example:
- Click Here To Show Diagram Code
[go]$$B
$$ ---------------------------------------
$$ | . . . . X X X X X X O . . . . . . . . |
$$ | . . O O X O O O O u O . . . . . . . . |
$$ | . . O X X X X X X X O . . . . . . . . |
$$ | . O O X X . O O O v O . . . . . . . . |
$$ | . O X X X X X X X X O . . . . . . . . |
$$ | . X X . X X O O O w O . . . . . . . . |
$$ | . X . . X X X X X X O . . . . . . . . |
$$ | X X . . . . . . . . . t . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . , . . . . . , . . . . . , . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . , . . . . . , . . . . . , . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ ---------------------------------------[/go]
the environment is the following:
Three gote points u, v, w with the values 4, 3½, 3 and I assume the remaining environment being an ideal environment at temperature t = 2½.
Can you find a better result than:
- Click Here To Show Diagram Code
[go]$$B
$$ ---------------------------------------
$$ | 5 2 7 8 X X X X X X O . . . . . . . . |
$$ | 4 1 O O X O O O O 6 O . . . . . . . . |
$$ | 3 . O X X X X X X X O . . . . . . . . |
$$ | . O O X X . O O O v O . . . . . . . . |
$$ | . O X X X X X X X X O . . . . . . . . |
$$ | . X X . X X O O O w O . . . . . . . . |
$$ | . X . . X X X X X X O . . . . . . . . |
$$ | X X . . . . . . . . . t . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . , . . . . . , . . . . . , . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . , . . . . . , . . . . . , . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ ---------------------------------------[/go]
- Click Here To Show Diagram Code
[go]$$Bm9
$$ ---------------------------------------
$$ | X 2 X O X X X X X X O . . . . . . . . |
$$ | 4 X O O X O O O O O O . . . . . . . . |
$$ | X 6 O X X X X X X X O . . . . . . . . |
$$ | 8 O O X X . O O O 3 O . . . . . . . . |
$$ | 1 O X X X X X X X X O . . . . . . . . |
$$ | 9 X X . X X O O O 5 O . . . . . . . . |
$$ | . X . . X X X X X X O . . . . . . . . |
$$ | X X . . . . . . . . . t . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . , . . . . . , . . . . . , . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . , . . . . . , . . . . . , . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ | . . . . . . . . . . . . . . . . . . . |
$$ ---------------------------------------[/go]
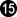
takes t/2
Many thanks, Gérard. You are always an inspiration.

I have studied this position a bit more, and I believe that I understand it better.

As always, I follow Berlekamp's dictum that to understand a position, start with its thermograph. The above position has the corner plus three simple gote with local temperatures greater than the temperatures of the ideal environment.
To understand the corner better, I made the three simple sente identical, with a temperature equal to that of the ideal environment, t = 4. IOW, we have the corner plus an ideal environment with 6½ ≥ t > 1.
In the sgf file below I compared these two lines of play with Black playing first.
1) Black plays first in the environment and then White plays in the corner, and then Black plays in the environment again.
2) Black starts the ko, which is sente. In the fight, with neither side having a ko threat, White plays once in the environment and Black plays twice, and then White plays in the corner to win the ko, and then Black plays in the environment again.
These two lines of play should produce the same net result. As the sgf file indicates, they do.

The three simple gote give leeway by adjusting their miai values for starting the ko to be the best play. What is intriguing is that values that create an environment that is very close to ideal does that.

Also, if it is White to play before the global temperature drops to 1, White will almost surely play in the environment. So Black will have many chances to gain from starting the ko at her turn. Well spotted, Gérard!









